OCR
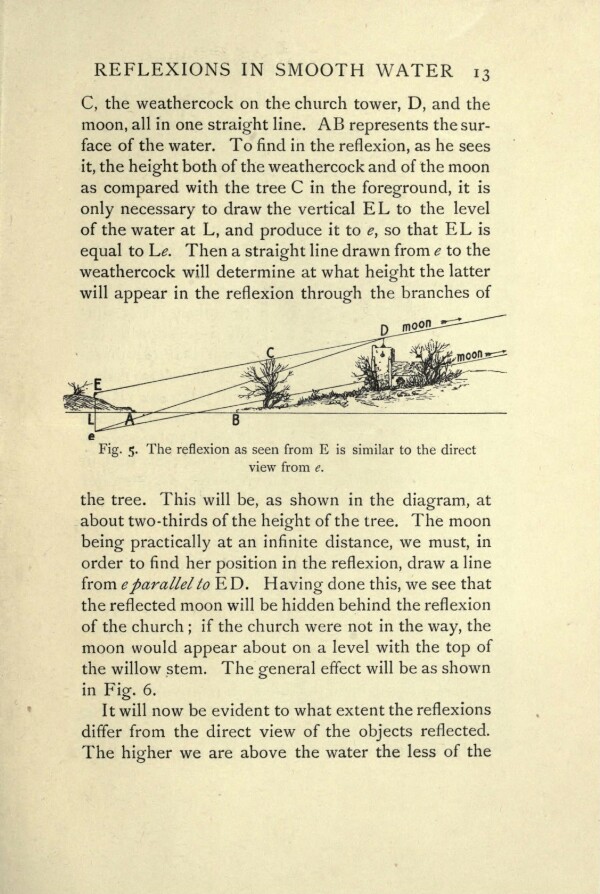
REFLEXIONS IN SMOOTH WATER 13 C, the weathercock on the church tower, D, and the moon, all in one straight line. AB represents the surface of the water. To find in the reflexion, as he sees it, the height both of the weathercock and of the moon as compared with the tree C in the foreground, it is only necessary to draw the vertical EL to the level of the water at L, and produce it to e, so that EL is equal to Le. Then a straight line drawn from e to the weathercock will determine at what height the latter will appear in the reflexion through the branches of the tree. This will be, as shown in the diagram, at about two-thirds of the height of the tree. The moon being practically at an infinite distance, we must, in order to find her position in the reflexion, draw a line from e parallel to ED. Having done this, we see that the reflected moon will be hidden behind the reflexion of the church; if the church were not in the way, the moon would appear about on a level with the top of the willow stem. The general effect will be as shown in Fig. 6. It will now be evident to what extent the reflexions differ from the direct view of the objects reflected. The higher we are above the water the less of the