Oldal 25 [25]
OCR
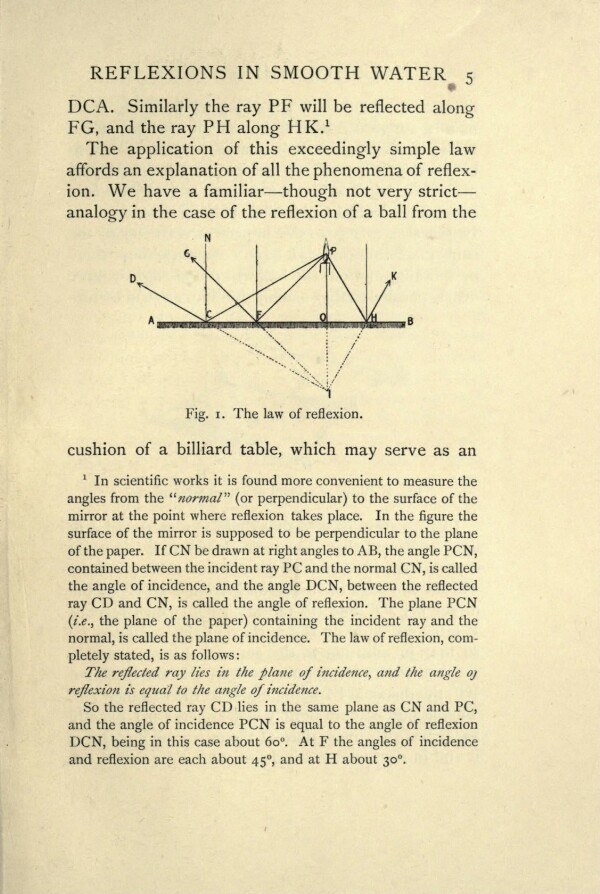
REFLEXIONS IN SMOOTH WATER : had DCA. Similarly the ray PF will be reflected along FG, and the ray PH along HK.’ The application of this exceedingly simple law affords an explanation of all the phenomena of reflexion. We have a familiar—though not very strict— analogy in the case of the reflexion of a ball from the D me kj ag . = \ *, a i Fig. 1. The law of reflexion. cushion of a billiard table, which may serve as an : In scientific works it is found more convenient to measure the angles from the “‘zormal” (or perpendicular) to the surface of the mirror at the point where reflexion takes place. In the figure the surface of the mirror is supposed to be perpendicular to the plane of the paper. If CN be drawn at right angles to AB, the angle PCN, contained between the incident ray PC and the normal CN, is called the angle of incidence, and the angle DCN, between the reflected ray CD and CN, 1s called the angle of reflexion. The plane PCN (z.e., the plane of the paper) containing the incident ray and the normal, is called the plane of incidence. The law of reflexion, completely stated, is as follows: The reflected ray lies tn the plane of incidence, and the angle oj reflexion ts equal to the angle of incidence. So the reflected ray CD lies in the same plane as CN and PC, and the angle of incidence PCN is equal to the angle of reflexion DCN, being in this case about 60°. At F the angles of incidence and reflexion are each about 45°, and at H about 30°.
